Parallel Line at a Given Distance

In Euclidean geometry, lines are said to be parallel if extended to infinity, the lines never meet. What would happen if you drew two parallel lines on the ground and extended them around the entire surface of the earth?

Scroll down to step through the instructions.

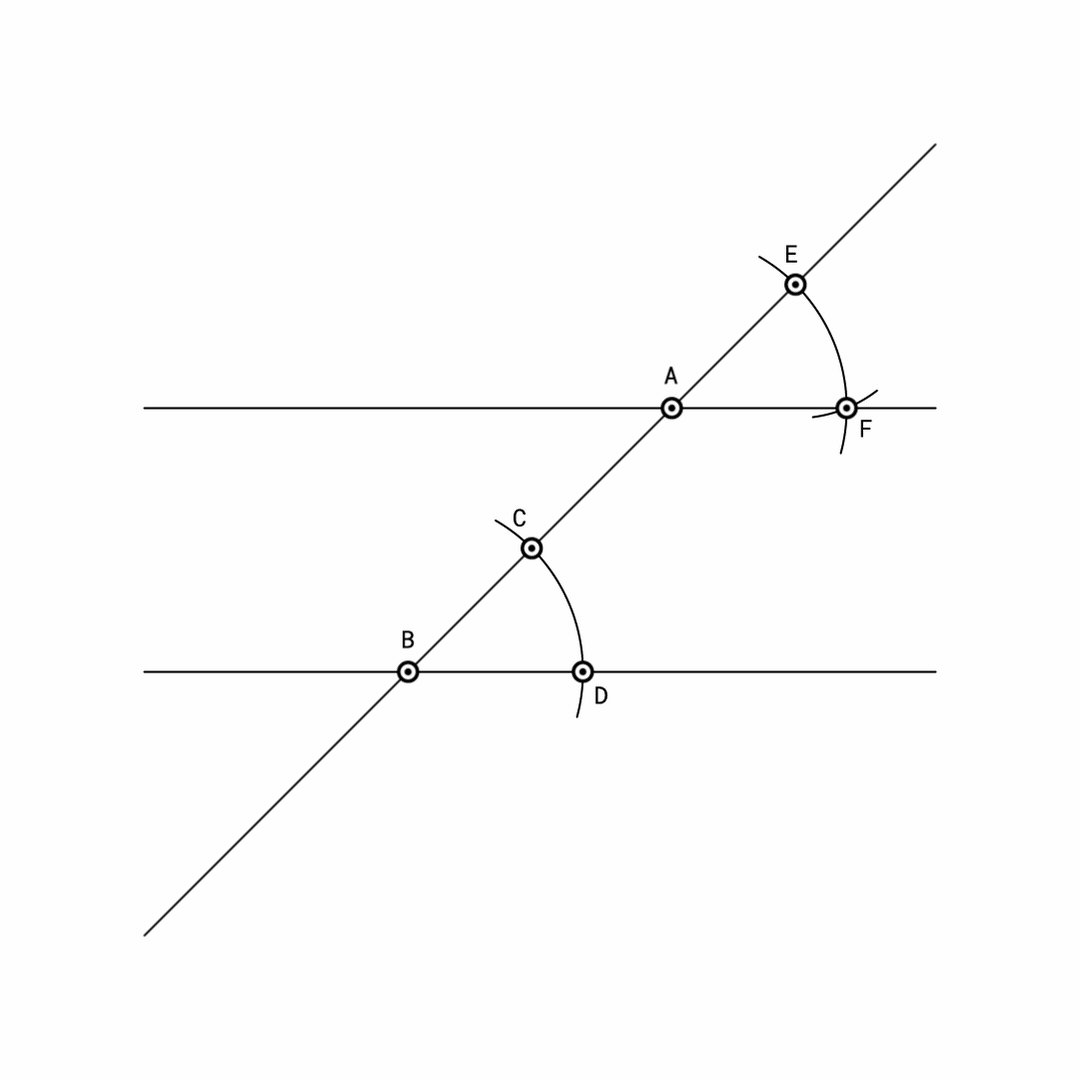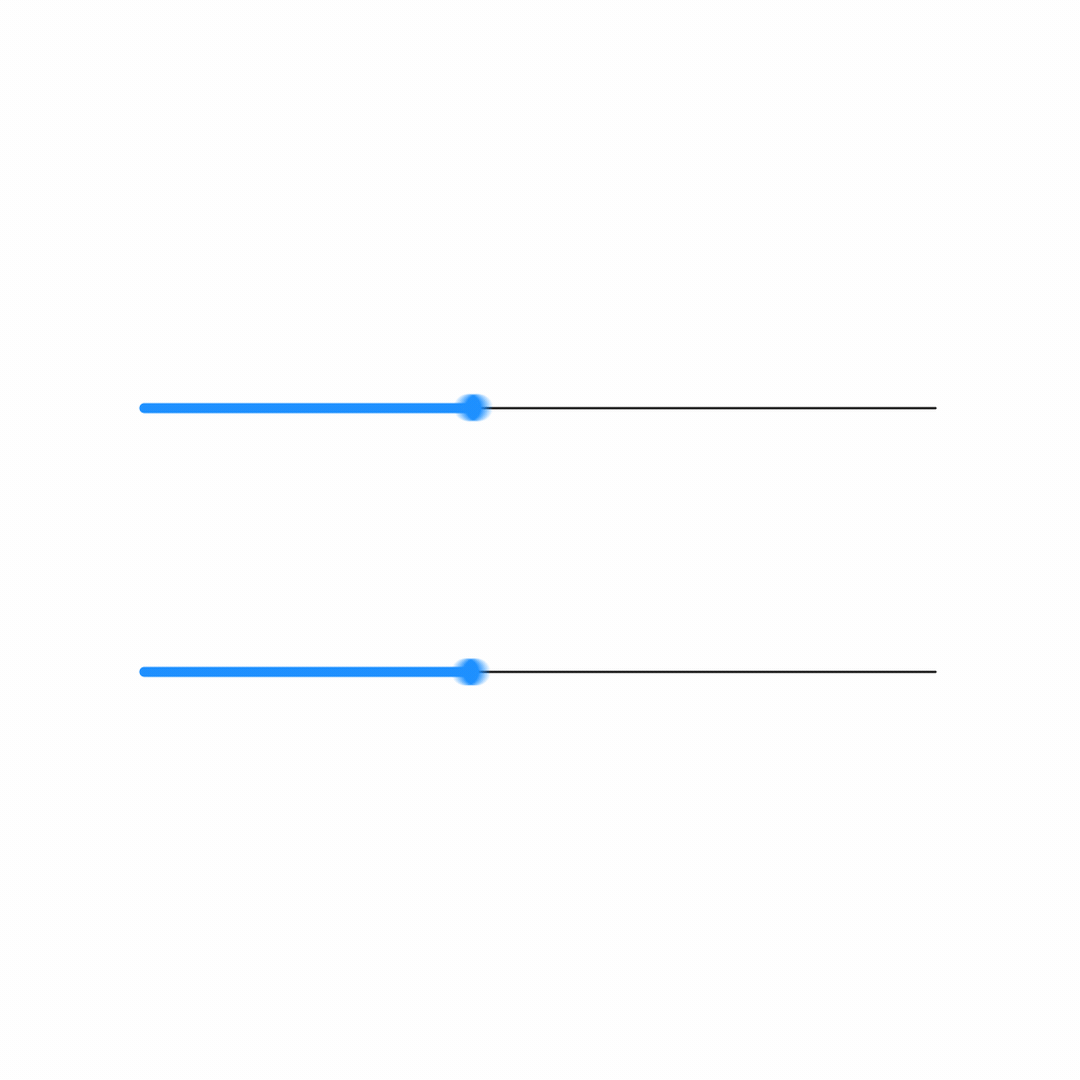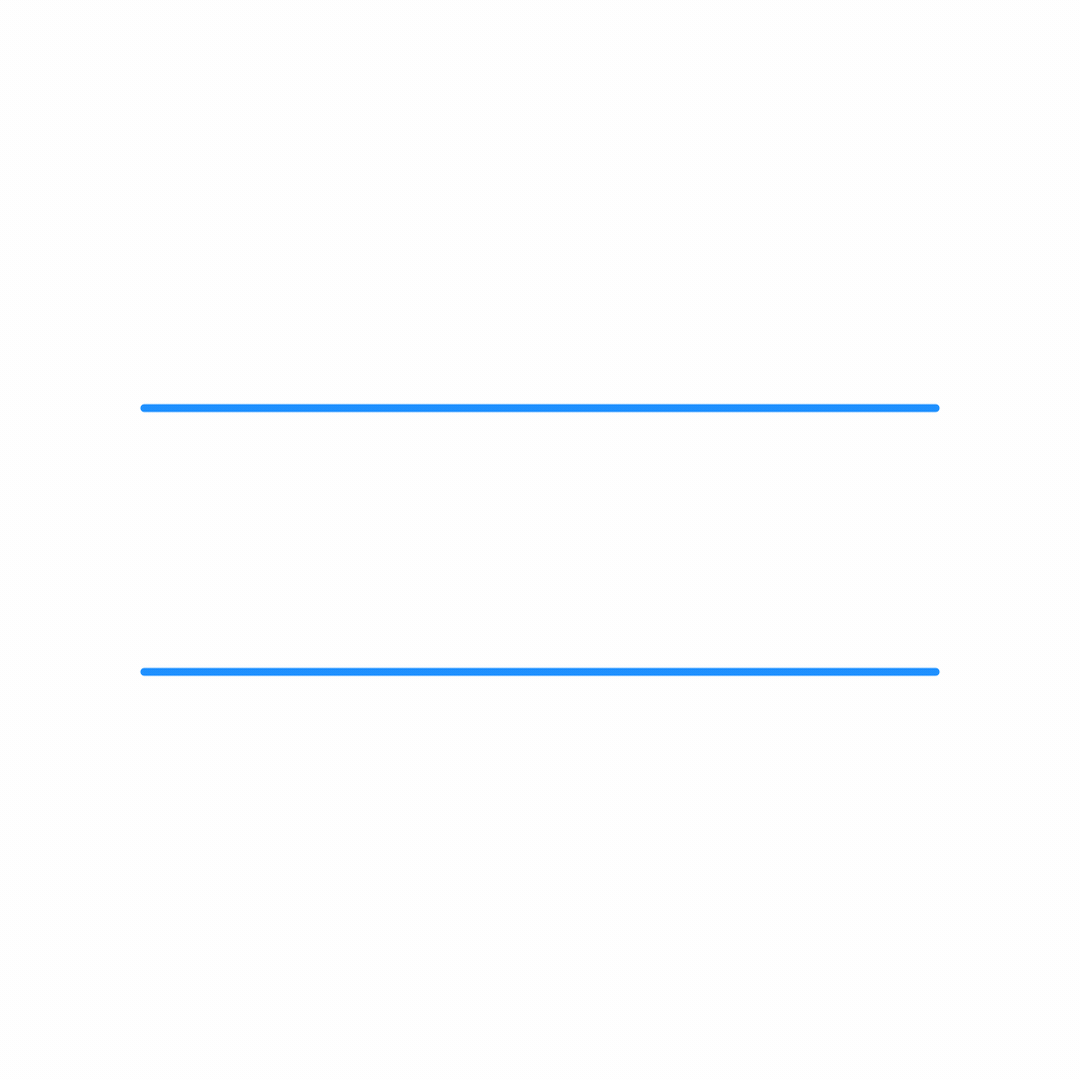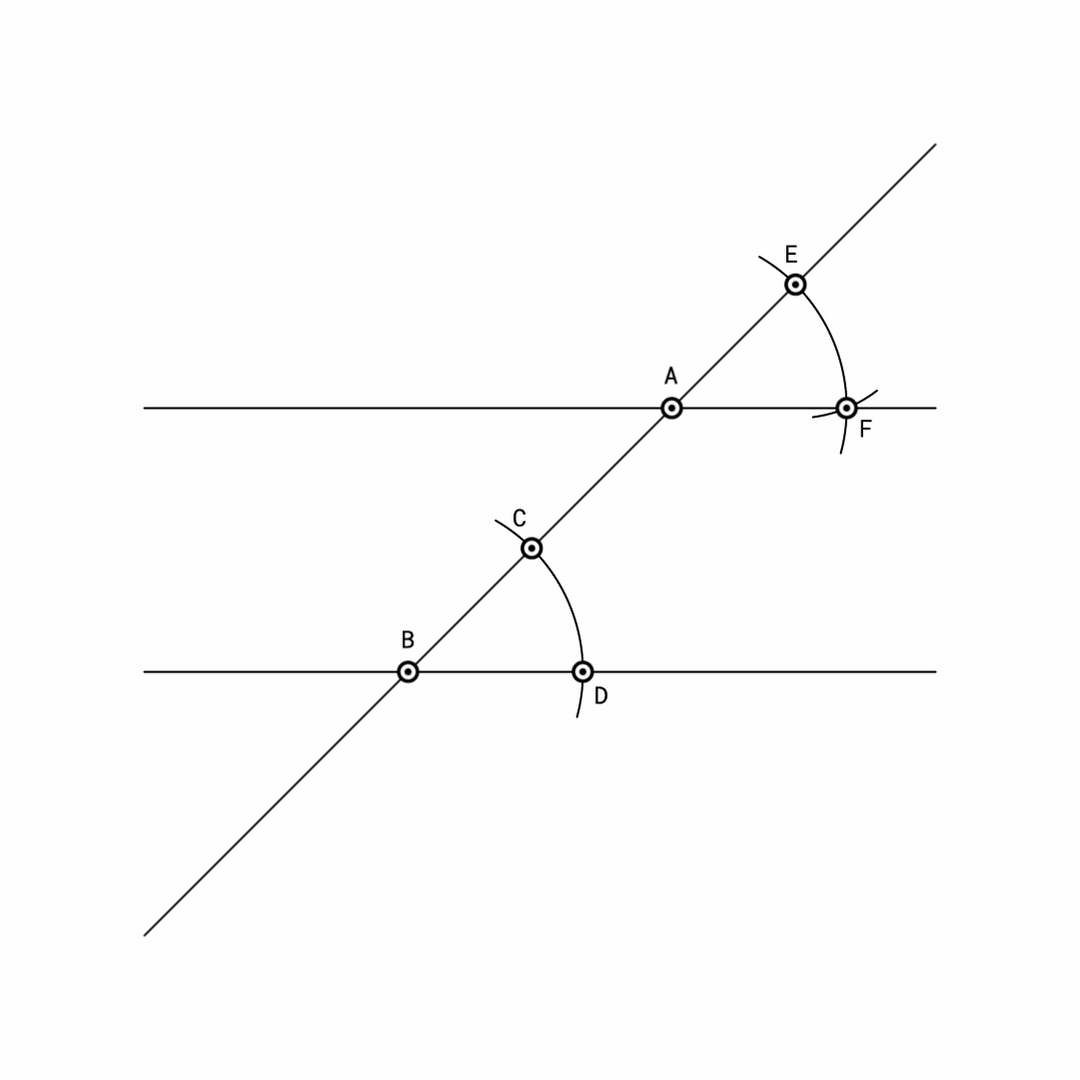
01. Start with a line and a point, which we will label A.

02. Select a point along your starting line, which we will label B.

Draw a line that passes through points A and B.

03. Draw an arc around point B that crosses both the starting line and line AB.

04. Draw an arc with the same radius around point A. This arc should cross line AB and also past where you expect a line parallel to the starting line to fall.

05. Identify the points of intersection between the arcs and lines and label as shown.

Using your string or rope, measure distance between points C and D.

Transfer that length of rope such that point C is now at point E.

Draw an arc around point E that intersects the arc around point A.

06. Identify the point of intersection of the arc around point A and the arc around point E and label it point F.

Draw a line that passes through points A and F.

07. You have drawn two parallel lines!